Projects
Project managment: PD Dr. habil. Anton Klimovsky with Prof. Dr. Lisa Hartung (Universität Mainz)
Funding institution: DFG
Funding code: 443891315, within priority programmeSPP 2265: Random geometric systems
Project period: 2020-2026
Project description: What is the effect of the space-time varying environment on the long time behavior of spatially structured populations of interacting particles/individuals/agents? This question is of high relevance, e.g., in life and social sciences/economics, computer science, artificial intelligence. Mathematically, the project focuses on two phenomenological models of interacting particle systems: (1) branching Brownian motion, which models population growth/spatial spreading; and (2) voter model, which models information exchange in a population of agents. The novel features that this project introduces into these classical models are: (1) space-time-correlated environments and (2) evolving networks. These play the role of the geographic spaces and substantially change the underlying spatial geometry. The project aims at investigating (1) growth vs. extinction, population size, spread; and (2) clustering vs. consensus of agents, space-time scaling limits of stochastic processes on evolving networks and evolving graph limits.
Project managment: Prof. Dr. Markus Bibinger
Employees: Malon Jansen, Michael Sonntag
Project period: 2018-2023
Funding institution: DFG
Funding code: 403176476
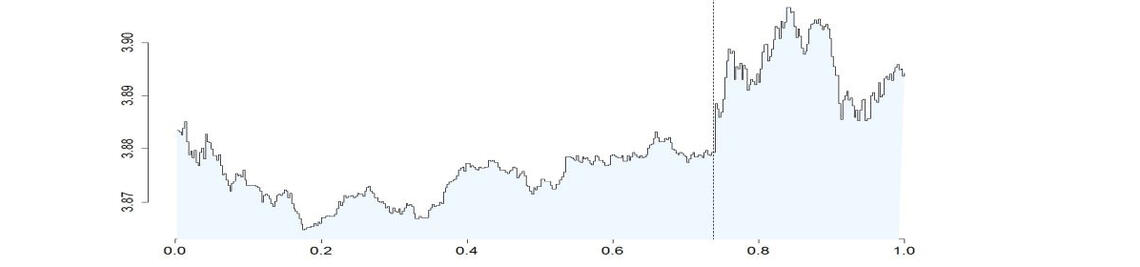
Project description: Volatility refers to the magnitude of random fluctuations in a system described by a stochastic differential equation. In recent years, considerable attention has been devoted to stochastic volatility processes. Their path properties determine, for instance, optimal volatility estimation methods, forecasting techniques and the volatility persistence. In this project, we develop statistical theory to infer path properties of volatility. Recently, there have been important contributions to advance estimation and testing procedures on path properties of stochastic processes from discrete observations. On the other hand, even though the path properties of volatility are of particular interest for applications, there is so far no groundwork on a statistical analysis. The key difference and difficulty is that volatility is latent and thus cannot be directly observed. Based on statistics involving pre-estimated volatility instead, Moritz Jirak and Markus Bibinger started to work in this new direction in a first article focusing on a change-point analysis. Exploiting recent contributions regarding volatility estimation from high-frequency data and inference on path properties from direct observations, we aim to establish a novel theory with optimal test and estimation approaches for path properties of the latent volatility. This is pursued for three related yet different observation models of interest. While one model assumes discrete observations of a semimartingale, the two others incorporate regular or non-regular observational noise. Our theory shall also explore the limitations of identifiability. While currently conflicting models for volatility processes are put forward in the literature, our methods aim to provide evidence which models are suitable. Our nonparametric statistical analysis reveals, moreover, if path properties are stable over time or changing. Particular interest in this work is motivated by statistics for financial markets. Volatility is the prevailing concept to describe market risk in price evolutions. Reliable volatility estimates are thus key ingredients for risk management and risk prediction. Our main focus is on intra-day high-frequency data at highest available recording frequencies. For many liquid financial instruments huge numbers of observations are nowadays available – usually several observations per second. Modeling and analysing such high-frequency financial data becomes more and more important as much volume, currently almost 70%, is attributed to high-frequency trading. At the same time, specific market frictions need to be taken into account, inducing noisy observations.
Project managment: PD Dr. habil. Anton Klimovsky
Funding institution: DFG
Funding code: 412848929
Project period: 2019-2022
Informationen: See website.
Project description: In the last 20 years, complex networks became a key tool to model real-world complex systems in the sciences. Yet, the majority of networks evolve over time and this can have a substantial effect on the processes unfolding on them. Moreover, the influence can also go the other way around: processes happening on a network can affect the evolution of the network itself leading to what is called coevolution in adaptive networks (or more generally in complex adaptive systems). Examples include epidemiological and ecological networks, neural networks, systems biology networks, social networks, financial markets, etc. In all these contexts, there is a great deal of uncertainty/volatility in the structure and dynamics of the complex system.What are the emerging global patterns in complex systems? How do they come about from the behavior of the elements? These are typical questions in the sciences, economy and policy making. These questions immediately lead to severe mathematical problems about the models of complex systems but also about their relationships with the real data.It is the purpose of this scientific network to advance the rigorous mathematical theory of stochastic processes on (co)evolving networks. The scientific network focuses on:(a) The probabilistic underpinnings of stochastic processes on (co)evolving networks.(b) Analysis and synthesis of key examples coming from the areas of information/opinion exchange processes, population dynamics, infection processes, and dynamics of artificial neural networks.(c) Issues of statistical inference, estimation and uncertainty quantification for (evolving) complex networks and processes on them.