Mathematical Physics (Bachelor)

Bachelor of science in Mathematical Physics

Degree:	Bachelor of Science
Target duration:	6 semesters
Academic term:	Winter semester
Admission	Free
Credits:	180 ECTS points
Target group:	Students who want to acquire a broad and solid foundation in mathematics and theoretical physics
Qualification targets:	Qualification targets Bachelor Mathematical Physics (180 ECTS)
Accreditation:	program accredited till September 30, 2028

Aspiring Mathematical Physicists should

become familiar with the mathematical foundations of theoretical physics
Get insights into experimental physics
acquire comprehensive knowledge in the field of theoretical physics
become independently capable to classify and solve questions of mathematical physics

A study of Mathematical Physics promotes

Ability related to abstract thinking
Skills to address complex issues
Perseverance and creativity in solving problems

Mathematics and Physics are two of the major sciences of our society, that are developed both for the sake of knowledge as well as for addressing direct applications in real life. Therefore, a general study of Mathematical Physics can provides an essential introductory knowledge that promotes

ability related to abstract thinking,
comprehensive knowledge in the methods of mathematics and physics,

as well as

Skills to address complex issues,
Ability to localize the essential elements of a problem,
Perseverance and creativity in solving problems.

This "complete package" implies excellent job prospects and the ticket to numerous fields of application in industry, science and research.

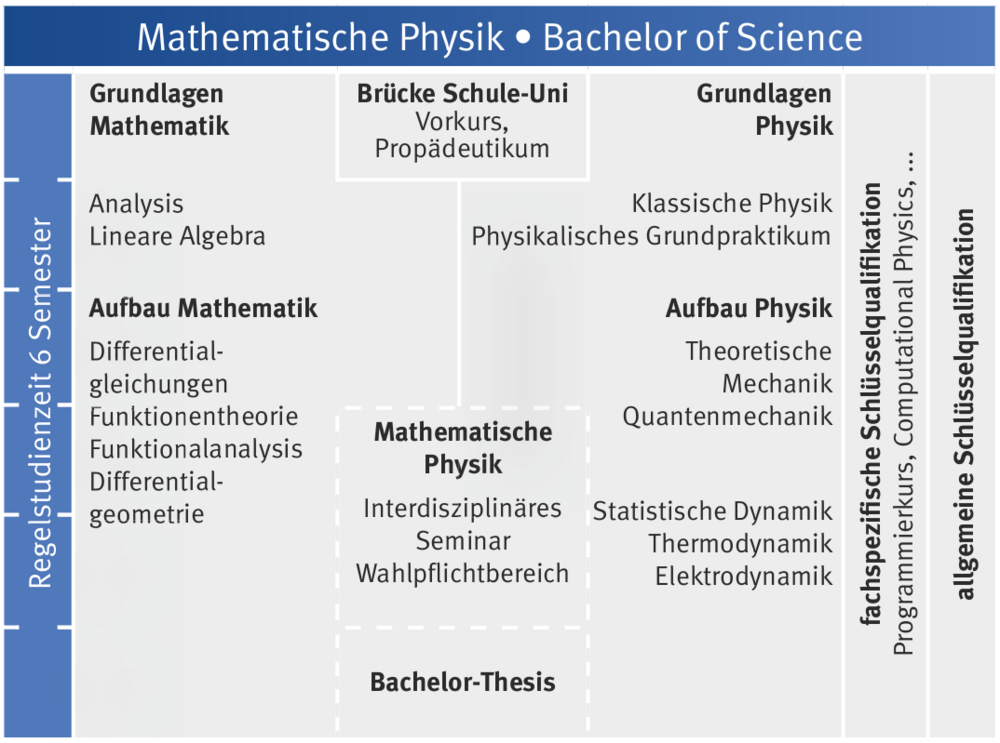
Structure

Analysis, Linear Algebra and Classical Physics each consist of Parts I and II. On the one hand the two courses of Mathematics are each completed with an oral exam; on the other hand two graded exams must be passed in Classical Physics. The basic physical element comprises several internships and events for error calculation. Further details on the areas of Mathematics, Physics and Mathematical Physics can be found below:

The Bachelor's degree program Mathematical Physics is divided into so-called modules, which include one or two lectures. Each module is completed with a final examination. Passed examinations are worth credit points (so-called ECTS), that eventually correspond to the required workload (1ECTS = 30 hours) (and should not be confused with grades).
You should attend lectures with exercises (usually 4 + 2 semester credit hours (SWS)), a seminar (2 SWS). In addition you should apply for an internship related to the physics program. Furthermore, there are subject-specific and general key qualifications (for example, programming or language courses) amounting to 20 ECTS.
In the first two semesters, with a curriculum that is essentially compulsory (Analysis, Linear Algebra, Classical Physics, and Basic Physics), you will study the basics of mathematics and physics.
In the second part of the study (semesters 3 to 6) you will attend lectures and seminars in the areas of mathematics, physics and mathematical physics. While the block of structural physics includes four compulsory courses in theoretical physics, the two other blocks are so-called elective areas, i.e. you can choose from different events.
Towards the end of your studies, you will prepare your written bachelor thesis, supervised by a lecturer, with a focus on mathematics or physics.

Examples of a study course plan (overview of modules to be completed)

Basics and Orientation exam

After the second semester, the successful completion of one of the modules Analysis I, Linear Algebra I, Classical Physics I or II allows to pass the orientation Examination. If this cannot be fulfilled (and only then), then the completion of one of the following modules must be provided after the third semester: General Overview of Analysis or Overall Overview of Linear Algebra as well as one of the modules Classical Physics I or II. If this cannot be done either, then the bachelor's degree program is considered failed.

Thesis

If you have earned 180 ECTS credits under the Examination Regulations, you will receive the academic degree of a Bachelor of Science.

Study and Examination Regulations

The examination board is u.a. responsible for

Recognition questions regarding achievements acquired in previous study programs / study locations
Recognition questions in the current study
Gender Equality

To the current cast

Overview of the modules

In the Bachelor's degree program Mathematical Physics there are the compulsory areas of Mathematics, Physics and Physics Internship. These include the following sub-modules for a total of 110 ECTS:

&nbs

Mandatory Mathematics (47 ECTS):
- Analysis 1 (8 ECTS)
- Gesamtüberblick Analysis (12 ECTS)
- Vertiefung Analysis (7 ECTS)
- Lineare Algebra 1 (8 ECTS)
- Gesamtüberblick Lineare Algabra (12 ECTS)
Compulsory Physics (48 ECTS):
- Klassische Physik 1 (8 ECTS)
- Klassische Physik 2 (8 ECTS)
- Theoretische Mechanik (5 ECTS)
- Quantenmechanik (5 ECTS)
- Übungen: Theoretische Mechanik und Quantenmechanik (6 ECTS)
- Statistische Physik und Elektrodynamik (6 ECTS)
- Übungen: Statischtische Physik (5 ECTS)
- Übungen: Elektrodynamik (5 ECTS)
Internship of Physics (15 ECTS)
- Praktikum A+B+C (3+4+4 ECTS)
- Fehlerrechnung (2 ECTS)
- Fortgeschrittene Fehlerrechnung und computergestütztes Arbeiten (2 ECTS)

The elective mathematics is divided into the subareas of Fundamentals of Mathematical Methods and Overall Overview of Mathematical Methods. Both of them should be covered for a total of 22 ECTS.

Basic mathematical methods (9 ECTS)
- Einführung in die Differentialgeometrie (9 ECTS)
- Gewöhnliche Differentialgleichungen (9 ECTS)
- Einführung in die Funktionentheorie (9 ECTS)
- Geometrische Analysis (9 ECTS)
- Einführung in die Funktionalanalysis (9 ECTS)
- Einführung in Partielle Differentialgleichungen (9 ECTS)
Overall Overview of Mathematical Methods (13 ECTS)
- Here are two different modules from the subsection Fundamentals Mathematical Methods combined to an oral exam. All combinations are allowed.

The compulsory elective Mathematical Physics is divided into the subcategories Supplement Mathematics, Experimental Physics, Supplementary Physics and Current Topics of Mathematical Physics. Overall, at least 18 ECTS must be achieved here.

Supplement mathematics
- Numerische Mathematik 1 (10 ECTS)
- Numerische Mathematik 2 (10 ECTS)
- Stochastik 1 (10 ECTS)
- Stochastik 2 (10 ECTS)
- Einführung in die Algebra (10 ECTS)
- Einführung in die Diskrete Mathematik (10 ECTS)
- Einführung in die Projektive Geometrie (10 ECTS)
- Einführung in die Zahlentheorie (10 ECTS)
- Operations Research
- Einführung in die Differentialgeometrie (10 ECTS)
- Gewöhnliche Differentialgleichungen (10 ECTS)
- Einführung in die Funktionentheorie (10 ECTS)
- Geometrische Analysis (10 ECTS)
- Einführung in die Funktionalanalysis (10 ECTS)
- Einführung in Partielle Differentialgleichungen (10 ECTS)
- Modellierung und Wissenschaftliches Rechnen (8 ECTS)
Experimental physics
- Optik und Wellen (8 ECTS)
- Atome und Quanten(8 ECTS)
- Einführung in die Festkörperphysik (8 ECTS)
- Kern- und Elementarteilchenphysik (6 ECTS)
Supplement physics
- Gruppentheorie (6 ECTS)
- Computational Physics (6 ECTS)
- Statistik, Datenanalyse und Computerphysik (4 ECTS)
- Atomphysik (6 ECTS)
- Teilchenphysik (8 ECTS)
Current topics of mathematical physics
- Aktuelle Themen der Mathematischen Physik (5 ECTS)
- Aktuelle Themen der Mathematischen Physik (6 ECTS)
- Aktuelle Themen der Mathematischen Physik (8 ECTS)

Modules from the Supplementary Mathematics subcategory that were already used in the elective module of Mathematics cannot be selected twice. There are no other restrictions.

The key qualifications (SQ) are divided into general SQ (5 ECTS) and subject-related SQ (15 ECTS).

With general SQ, you can choose from a large selection of suitable modules from all faculties. Very popular are for instance Language courses as well as tutor and corrector activities in mathematics (from the 3rd semester). The current list can be found on this page.

The subject-related SQs are further subdivided into

compulsory modules (9 ECTS)
- pre course (2 ECTS)
- preparatory course (2 ECTS)
- seminars of Mathematical Physics (5 ECTS)
Elective Modules (6 ECTS)
- Ergänzungsseminar Mathematik (4 ECTS)
- Einführung in die Topologie (5 ECTS)
- Computerorientierte Mathematik (4 ECTS)
- Programmierkurs (3 ECTS)
- Geschichte der Mathematik (5 ECTS)
- Mathematisches Schreiben (5 ECTS)
- Schulmathematik vom höheren Standpunkt (4 ECTS)
- Proseminar (4 ECTS)
- Mathematische Rechenmethoden Physik (6 ECTS)
- Computational Physics (6 ECTS)

Thesis

The bachelor thesis (BAT, 11 ECTS) is the last major examination in your bachelor program. This written work is designed to show that you are capable of scientifically handling a (mathematical) problem within a set period of ten weeks. No scientific new value is expected from you, but just a scientific approach.

At the very latest you should start with your BAT ten weeks before the end of your 8th semester, so that you can still use the full processing time to complete the work before the beginning of your 9th semester (with the beginning of the 9th semester, your Bachelor degree program is considered not completed for the first time!).

In order to get a topic for the BAT, you can (at first) be informed at the bulletin boards in front of the Mathematics Branch Library: there, current topic suggestions depend on different areas of mathematics and different supervisors.

If you do not find it there, you should personally contact a lecturer in mathematics according to your mathematical interests (see Overview of the Chairs of the Institute of Mathematics) and define a topic directly with him / her (your own suggestions are possible!). Potential supervisors are all lecturers in mathematics.

If you have found a supervisor and a subject for your BAT, please print out this Form, fill it out together with your supervisor and give it to Ms. Schmid (Mathematik Ost, Zi. 00.016). This will make the topic of your BAT and the date of submission (date of issue of the topic + 10 weeks) official and binding.

Only in well justified cases (incapacity for examination, with attest!) The processing time can be extended upon application to the examination board and in consultation with the supervisor. The BAT should be printed twice as well as it should be saved on a electronic storage medium "in a common format and in readable form" (quote ASPO, pdf file on a data CD is a viable option). The Thesis should be handed in time to the examination office, specifically to Mrs. Feineis. There, the adherence to the processing time is documented and your work is passed on to the designated referee.

Does Mathematical Physics fit me?

If you want to understand mathematics and physics - especially theoretical physics - then you are in the right place - otherwise we recommend an advisory discussion.